4 - Economic applications

Prev Next

Simultaneous Equations

Section 4 Some examples of simultaneous equations in economics

Students who are not familiar with the basic terms and concepts in economics, are recommended to read only Section 1, 2 and 3 and leave Section 4 for a suitable time/ when required. In addition to reading this chapter, we recommend to practice from a suggested book.

Goods Market Equilibrium

Example 1

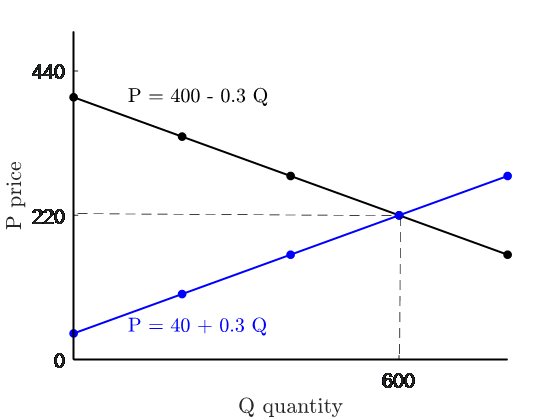
The inverse demand and supply functions for a commodity are

$$\text{Inverse demand function: } P_{d} = 400 - 0.3Q$$ $$\text{Inverse supply function: } P_{s} = 40 + 0.3Q \quad\,$$ Where, $P$ shows the market price and $Q$ shows the quantity. Subscript $d$ represents demand and subscript $s$ represents the supply. Calculate the equilibrium price.

Equilibrium price is the price that makes the demand and supply exactly equal. In a static equilibrium, it requires that in the equilibrium $P_d= P_S$. $$400 - 0.3Q = 40 + 0.3Q$$ $$0.6Q = 360$$ $$Q = 600$$ Now solve for the equilibrium $P$ by substituting $Q=600$ into either the demand or the supply equation $$P = 400 - 0.3(600) = 220$$

Figure 5 shows the inverse demand and supply functions and their intersection point at $(600, 220)$.

Figure 5

Market equilibrium for the substitute and complementary goods

Complementary goods (for example, cars and gasoline) are those that the consumer consumes together; substitute goods are those that the consumer consumes in place of one another (for example, coffee and tea).
The general demand function including the price of a related good is: $$Q = f(P, P_{r}, I)$$ That is, the quantity demanded of a good $Q$ is a function of its own price $(P)$, prices of related goods $(P_r )$ and consumer income $(I)$.

Example 2

Given the following set of simultaneous equations for two related goods, $x$ and $y$, find the equilibrium conditions for each market if the consumers’ income is $I=600$. What types of goods are $x$ and $y$? $$Q_{dx} = 22 - 3P_{x} + P_{y} + 0.1I$$ $$Q_{sx} = -5 + 15P_{x} \qquad\qquad\;$$ And $$Q_{dy} = 32 + 2P_{x} - 4P_{y} + 0.1I$$ $$Q_{sy} = -6 + 32P_{y} \qquad\qquad\;\;\;$$

By replacing $I=600$ and setting $Q_{dx}=Q_{sx}$ we get: $$82 - 3P_{x} + P_{y} = -5 + 15P_{x}$$ $$\qquad\quad\;\; 18P_{x} - P_{y} = 87 \qquad\qquad\;(1)$$

By replacing $I=600$ and setting $Q_{dy}=Q_{sy}$ we get: $$92 + 2P_{x} - 4P_{y} = -6 + 32P_{y}$$ $$\qquad\quad -2P_{x} + 36P_{y} = 98 \qquad\qquad(2)$$

From equation (1) we can solve for $P_y$ in terms of $P_x$: $$\qquad\qquad\;\; P_{y} = 18P_{x} - 87 \qquad\qquad(3)$$

Substitute $P_y=18P_x-87$ in equation (2): $$-2P_{x} + 36(18P_{x} - 87) = 98$$ $$\qquad\qquad\qquad\qquad\; 646P_{x} = 3230$$ $$\qquad\qquad\qquad\qquad\; P_{x} = 5$$

Similarly, we can get $P_y=3$, $Q_x=70,Q_y=90$

These two goods are substitute goods. Consider the demand equation $Q_{dx}=22-3P_x+$$P_y$$+0.1 I$; here, the coefficient of $P_y$ is positive. This means, if the price of the related good ($P_y$) increases, the quantity demanded for the good $x$ ($Q_{dX}$) also increases. Now consider the demand function $Q_{dy}=32+2P_x$$-4$$P_y+0.1 I$; we see that the coefficient of $P_y$ is negative in its own demand function. This means, if $P_y$ increases, $Q_{dy}$ decreases. What we see from these two demand equations is that when the demand for $y$ decreases, the demand for $x$ goes up. So, in this example, these two goods are substitute goods. [To see more examples about substitute goods and complementary goods, see Dowling $T$, Mathematical Economics, Chapter 2.]

Revenue and cost functions

Example 3

Assume that a firm can sell all its products it manufactures in a month at $$25$ each. It has to pay out $$300$ fixed costs. Also, the firm must pay a marginal cost of $$20$ for each unit produced. How much does the firm need to produce to earn zero profit (break-even)?

The total revenue function $(TR)$ of the firm is the price of the product $($25)$ multiplied by the quantity sold $(x)$: $$TR = 25x$$ To produce $x$ units, the firm must pay out $$300$ fixed costs and a marginal cost of $$20$ for each unit. Hence, the firm has the total cost function $(TC)$: $$TC = 300 + 20x$$ When its total revenue equals the total cost, the firm earns zero profit which is the break-even point for the firm $$TR = TC$$ $$\qquad\;\;\; 25x = 300 + 20x$$ $$25x - 20x = 300 \qquad\;\;$$ $$\;\;\;5x = 300$$ $$\;\;\;x = 60$$

National income model

National income models express the equilibrium level of income generally as $$Y = C + I + G + (X - Z)$$ Where $Y$ is the aggregate income generated in the economy from aggregate consumption $(C)$, investment $(I)$, government expenditure $(G)$, and net export (export $(X)$ minus import $(Z)$), generally measured in a year. Alternatively, $Y$ is the total income and $C+I+G+(X-Z)$ is the total expenditure $(E)$ in the economy in a year and the equilibrium national income occurs when the total income $(Y)$ and total expenditure $(E)$ are equal. If all equations are linear in a national income model with $m$ sectors, we can solve the system using the method of substitution to find equilibrium values for the endogenous variables such as, aggregate income, aggregate consumption etc. In example 4, we discuss a simple economy with three sectors, but the method can be applied to as many sectors as are available, given that all equations are linear and consistent.

Example 4

Assume a simple three-sector economy where $$Y = C + I + G$$ $$C = C_{0} + bY \;\;\;$$ $$I = I_{0} + aY \;\;\;$$ $$G = G_{0} \qquad\quad$$ Here, $C_0, I_0, G_0, a$ and $b$ are given with certain economic restrictions
Find the equilibrium income $(Y)$ and consumption $(C)$.

$$Y = C + I + G$$ Substitute for $C$ $$Y = C_{0} + bY + I + G$$ Substitute for $I$ $$Y = C_{0} + bY + I_{0} + aY + G$$ Substitute for $G$ $$Y = C_{0} + bY + I_{0} + aY + G_{0}$$ Solving for $Y$ $$Y - bY - aY = C_{0} + I_{0} + G_{0}$$ $$Y(1 - b - a) = C_{0} + I_{0} + G_{0}$$ $$\qquad\qquad\qquad\qquad\qquad Y = \frac{1}{1 - b - a}(C_{0} + I_{0} + G_{0})$$ Substituting equilibrium income in the consumption function, we can derive the equilibrium level of aggregate consumption.

In national income accounting, ${{1}\over {(1-b-a)}}$ is called the multiplier and $C_0+I_0+G_0$ is the autonomous expenditure. The multiplier measures the multiple effect of each dollar of autonomous expenditure on the equilibrium level of national income. The restrictions necessary on the constant terms in the model are $0 < a < 1$, $0 < b < 1$, and $0 < a+b < 1$ which ensures that the multiplier is greater than $1$.

To give an example of the multiplier, if $b=0.7$ and $a=0.1$, then the multiplier is ${{1}\over {(1-b-a)}}={{1}\over {(1-0.7-0.1)}}=5$ which means that if the autonomous expenditure increases by $$100$, the equilibrium income increases by $5×$100=$500$.

To read more about autonomous expenditure and multiplier, etc. read more about national income accounting. Recommended books contain a few examples from the national income accounting model.

Example 5

Assume a simple three-sector economy where $$Y = C + I + G$$ $$C = C_{0} + bY \;\;\;$$ $$I = I_{0} + aY \;\;\;$$ $$G = G_{0} \qquad\quad$$ Where $C_{0} = 65$, $I_{0} = 50$, $G_{0} = 20$, $b = 0.7$, $a = 0.1$
Find the equilibrium income $(Y)$ and consumption $(C)$.