Section 2

The derivative

Suppose \(y = f(x)\). If \(x\) changes from \(x_{0}\) to \(x_{1}\), the change in \(x\) is, $$\Delta x = x_{1} - x_{0}$$ and the corresponding change in \(y\) is, $$\Delta y = y_{1} - y_{0} = f(x_{1}) - f(x_{0})$$ Then, $$\frac{\Delta y}{\Delta x} = \frac{f(x_{1}) - f(x_{0})}{x_{1} - x_{0}}$$ is called the difference quotient which measures the average rate of change of \(f(x)\) with respect to x as we move from \(x_{0}\) to \(x_{1}\). Graphically it is interpreted as the slope of the secant line AB in Figure 2.

If the change in \(x \;\; (= \; \Delta x)\) tends to zero, we write the \(\Delta x\) as \(dx\) and the corresponding change in \(y\) as \(dy\) (instead of \(\Delta y\)), and the rate of change in \(y\) with respect to the very small change in \(x\) becomes

\(\frac{df(x_{0}}{dx}\) or simply \({f}'(x_{0})\)

Which is called the derivative of the function with respect to \(x\) at the point \((x_{0}, f(x_{0}))\). This can be interpreted as the slope of the tangent line at point A in Figure 2, or the slope of the function at A.

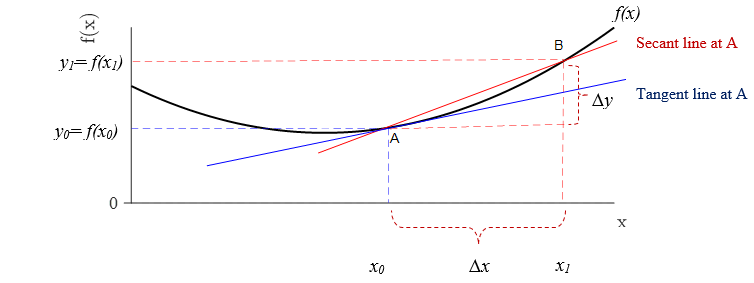
It’s worth mentioning here that a necessary condition for a function to be differentiable at a point is the continuity of the function at that point; i.e., to be differentiable at a point, the function must be continuous at that point. However, continuity of the function does not guarantee that the function is differentiable at that point. There are functions continuous at a point, yet not differentiable at that point. To read more about the continuity and differentiability, please see section 4 and please read Mathematics for Economists by Simon and Blume. The function depicted in Figure 2 is continuous and differentiable at point A.