6 - Uses

Prev Next

Derivatives and their uses

Section 6 Uses of the derivatives

Increasing and decreasing functions

Using the first-order derivative of a function $f(x)$ evaluated at $x = a$ , we can find whether the function is increasing or decreasing at an open interval containing $a$, or whether the point is a critical point.

If the first-order derivative evaluated at $a$ is positive, i.e. ${f}'(a) > 0$, then there is an open interval containing $a$ on which the function is increasing. If the first-order derivative evaluated at $a$ is negative, i.e. ${f}'(a) < 0$, then there is an open interval containing $a$ on which the function is decreasing. If the first-order derivative of the function at point $a$ is zero, i.e. ${f}'(a) = 0$ or undefined, then the point $x = a$ is called the critical point.

Open and closed intervals:

Given two real numbers $c$ and $d$, the set of all numbers between $c$ and $d$ is an interval. If the interval excludes the endpoints $c$ and $d$, the interval is an open interval which is written as $(c,d)$. On the other hand, $[c,d]$ is a closed interval as the interval includes the endpoints $c$ and $d$.

For example, consider a function $f(x) = x^{3} - 12x$. First-order derivative of this given function ${f}'(x) = 3x^{2} - 12$ carries much information about some important properties of the function. For example, there is an open interval containing $3$ at which the function is increasing as ${f}'(3) > 0$. For the same function, there is an open interval containing $1$ at which the function is decreasing which is evident from the fact that ${f}'(1) < 0$. The given function has two critical points at which ${f}'(x) = 0$. These critical points are $x = 2$ and $x = -2$.

Concave and convex functions

Second-order derivative evaluated at $x = a$ can be useful to find whether the function is concave or convex at an open interval containing $a$. If ${f}''(a) > 0$ for a differentiable function, there is an open interval containing $a$ at which the function is convex. If ${f}''(a) < 0$ for a differentiable function, there is an open interval containing a at which the function is concave. For example, consider the function $f(x) = x^{3} - 12x$. At the immediate neighborhood near the point $x = 2$ the function is convex which is supported by the fact that ${f}''(2) > 0$. As ${f}''(-2) < 0$ for the same function, at the immediate vicinity of $x = -2$, the function is concave.

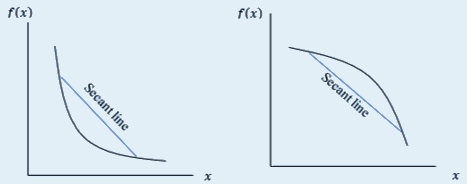
Non-calculus definition of concave and convex functions:For a function which is convex in an interval, the secant line joining any two points on that interval lies above the graph. If the secant line joining any two points in an interval lies below the graph, the graph is concave in that interval. See Figure 5 (a) and 5(b).

Figure 5(a) Convex function Figure 5(b) Concave function

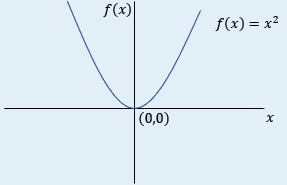
Knowing where a function is convex, or concave is a valuable piece of information for sketching the graph. For example, consider the function $f(x) = x^{2}$. It has a critical point at $x = 0$ where the function is neither increasing, nor decreasing. The second-order derivative of the function, ${f}''(x) = 2 > 0$ confirms that the function is convex at the neighbourhood of $x = 0$ . Using these two properties, we draw the graph of the function at Figure 6.

Figure 6

Inflection points

For a function $f(x)$ if there exist a point where ${f}''(a) = 0$ which divides the domain in two intervals around the point $a$ on one of which the second-order derivative is strictly positive and on another – strictly negative, the point is called the inflection point. At the inflection point, the sign of the second-order derivative changes.

Consider the function $f(x) = x^{3} - 12x$. At $x = 0, {f}''(0) = 0$. At $x = 0$, the domain is divided into two intervals. In the left of $x = 0, {f}''(x) < 0$. At another interval which is on the right of $x = 0, {f}''(x) > 0$. Therefore, in this example, $x = 0$ is an inflection point.

Maxima and minima

Another beneficial use of the derivatives is to find maxima and minima of functions, if any exist. For example, if the domain is unrestricted, i.e., $x$ can take any value on a real line, the function $y = 10 + 2x$ has no maxima or minima. However, many functions may have maxima or minima or both on their respective domains. For example, the function $f(x) = x^{3} - 12x$ has a local maximum (max) which hereafter will be simply called maximum (or max) and a local minimum (min) which hereafter will be simply called minimum (or min) on its unrestricted domain.

Consider a function $f$. Suppose there is an open interval in the domain of the function containing $a$ for which $f(x) \leq f(a)$ for all $x$ on that interval. Then, $a$ is a local or relative maximum of the function $f$. Consider another function $f$. Suppose there is an open interval in the domain of the function containing $a$ for which $f(x) \geq f(a)$ for all $x$ on that interval. Then, $a$ is a local or relative minimum of the function $f$.

We may also get the max or min of a function at the boundary points of the domain. In such cases, we call the max or min as the boundary max or boundary min. For example, if $y = 2x$, with the domain is a closed interval $[0,2]$, (i) at $x = 0$ we get the boundary min, and (ii) at $x = 2$ we get the boundary max. If the domain is unrestricted or open for the function $y = 2x$, we cannot obtain any max or min.

If we get a max or min which is not at any boundary of the domain, the max or min is the interior max or interior min, and we call them max or min. $f(x) = x^{3} - 12x$ has an interior min at $x = 2$ and an interior max at $x = -2$.

From now onward in this chapter, we will discuss examples which may only have interior max or min. But please remember that in intermediate economics courses you will frequently see examples with boundary max or min or an optimum at a corner point.

If $a$ is an interior critical point of a function and the function is differentiable at $a$, we can use calculus to decide whether the critical point is a max, min or neither.

If ${f}'(a) = 0$ and ${f}''(a) < 0$ then $a$ is a max of $f$.
If ${f}'(a) = 0$ and ${f}''(a) > 0$ then $a$ is a min of $f$.
If ${f}'(a) = 0$ and ${f}''(a) = 0$ then $a$ can be a max, a min, or neither.

If $f(x)$ is a differentiable function:

First-order necessary condition to be an interior critical point: ${f}'(x) = 0$

Second-order sufficient conditions:
If ${f}'(a) = 0$

${f}''(a) < 0$ implies that the $f(a)$ is a max of $f$.
${f}''(a) > 0$ implies that the $f(a)$ is a min of $f$.
${f}''(a) = 0$ implies that the $f(a)$ can be a max, min, or neither.

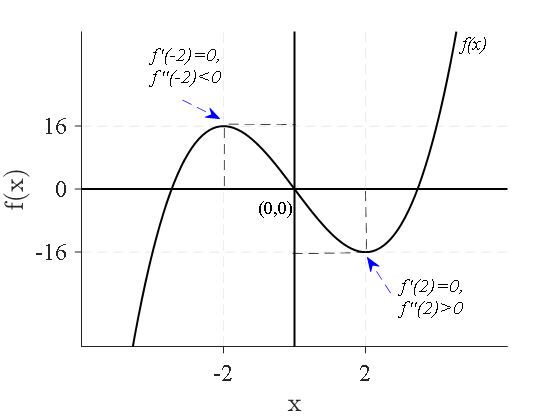
Example 1: See the following properties of the function $f(x) = x^{3} - 12x$.

${f}'(x) = 3x^{2} - 12$
${f}'(3) = 3(3)^{2} - 12 = 15 > 0$ Increasing on an open interval containing 3
${f}'(1) = 3(1)^{2} - 12 = -9 < 0$ Decreasing on an open interval containing 1
${f}'(x) = 3(x)^{2} - 12 = 0 \Rightarrow x \pm 2$ Critical points
${f}''(x) = 6x$
${f}''(2) = 6(2) = 12 > 0$ Convex around the neighbourhood of $2$
${f}''(-2) = 6(-2) = -12 < 0$ Concave around the neighbourhood of $-2$
${f}'(2) = 0$, ${f}''(2) > 0$ Local min
${f}'(-2) = 0$, ${f}''(-2) < 0$ Local max

We draw the graph of $f(x) = x^{3} - 12x$ using the above properties in Figure 7.

Figure 7

Uses of the derivatives: key points

Let us summarize our discussion about the uses of the derivatives:

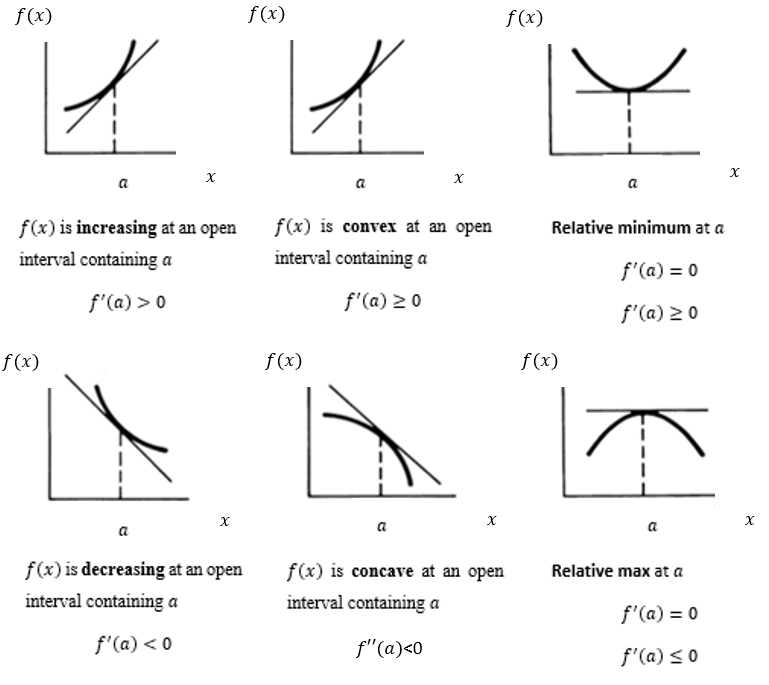
Suppose that the function $y = f(x)$ is continuously differentiable at $x = a$. Then there is an open interval containing $a$ on which the function is:

Increasing if ${f}'(a) > 0$
Decreasing if ${f}'(a) < 0$
Convex if ${f}''(a) > 0$
Concave if ${f}''(a) < 0$

Moreover, the function is

Neither increasing nor decreasing if ${f}'(a) = 0$
At a relative maximum if ${f}'(a) = 0$ and ${f}''(a) < 0$
At a relative minimum if ${f}'(a) = 0$ and ${f}''(a) > 0$
At an inflection point (concavity changes) if ${f}''(a) = 0$, and the concavity changes at $a$

Using these properties, we can draw several functions.

Figure 8

Example 2

Given $f(x) = 12x - x^{2}$, use the first-order derivative and the second-order derivative to sketch the graph of the function.

Here, ${f}'(x) = 12 - 2x$ and ${f}''(x) = -2$.

Using the properties obtained from its derivatives, we can find the intervals where the function is increasing, decreasing, concave and (or) convex. We can also see the inflection points if any. Using the derivatives, we can also find the critical points and the corresponding extreme points of the function, if available. For the given function $f(x) = 12x - x^{2}$, derivatives can provide us many important information such as,

As ${f}'(2) = 12 - 4 = 8 > 0$, there is an open interval containing $x = 2$, where the function is increasing.
As ${f}'(6) = 12 - 12 = 0$, at $x = 6$ the function is neither increasing nor decreasing.
As ${f}'(8) = 12 - 16 = -4 < 0$, there is an open interval containing $x = 8$ where the function is increasing.
At ${f}'(x) = 0$ we obtain the critical points of the function, if any. In this function, at $x = 6$, ${f}'(6) = 0$, which suggests that $x = 6$ is the only critical point for the function.
Second-order derivative of the function evaluated at the critical point can confirm whether the critical point is a max, min or neither. For the given function ${f}''(6) = -2$ confirms that near the vicinity of the critical point the function is concave. That means, the critical point $x = 6$ is a maximum point for the function.
To sum up:
At $x = 6$, ${f}'(6) = 0, {f}''(6) = -2 < 0$, the function is at a relative maximum
Finding the values of the function at $x = 0$, and at the critical point is also useful to sketch the graph:
At $x = 0$, $f(x) = 0$ vertical intercept $(0,0)$
At $x = 6$, $f(6) = 36$ maximum point of the function
The function has no inflection point as the second order derivative ${f}''(x) = -2$ can never be zero and can never change the sign of the second-order derivative from negative to positive.

Using the above properties of the function, we sketch the function in Figure 9.

(Try to relate the following graph of the function with a familiar function in economics. We will discuss it later.)

Figure 9

Example 3

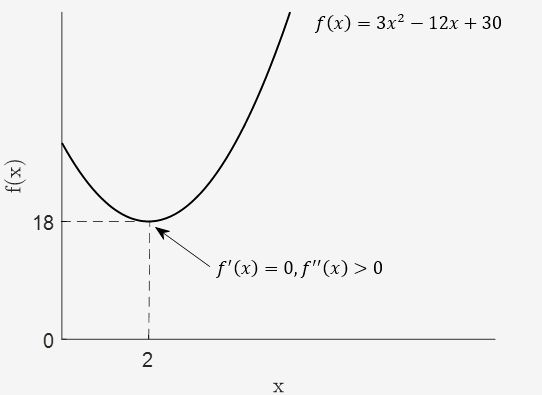
Given $f(x) = 3x^{2} - 12x + 30$, use the first-order derivative and the second-order derivative to sketch the graph of the function.

Here, ${f}'(x) = 6x - 12$ and ${f}''(x) = 6$.

To sketch the graph of the function using the properties obtain from its derivatives, we need to find the intervals where the function is increasing, decreasing, concave and (or) convex. We can also find the inflection points if any. Using the derivatives, we can also find the critical points and the corresponding extreme points of the function, if available.

As ${f}'(1) = 6 - 12 = -6 < 0$, there is an open interval containing $x = 1$, where the function is decreasing.
As ${f}'(2) = 12 - 12 = 0$, at $x = 2$ the function is neither increasing nor decreasing.
As ${f}'(3) = 18 - 12 = 6 > 0$, there is an open interval containing $x = 3$ where the function is increasing.
Critical points of the function are there where ${f}'(x) = 0$. In this function, at $x = 2$, ${f}'(2) = 0$ which suggests that $x = 2$ is the only critical point.
Second-derivative of the function evaluated at the critical point can confirm whether the critical point is a max, min or neither. For the given function ${f}''(2) = 6$ confirms that near the vicinity of the critical point the shape of the function is convex. That means, the critical point $x = 2$ is a minimum point for the function.
To sum up:
At $x = 2$, ${f}'(x) = 0, {f}''(x) = 6 > 0$, the function is at a relative minimum
Finding the values of the function at $x = 0$, and at the critical point, is also useful to sketch the graph:
At $x = 0$, $f(0) = 30$ vertical intercept $(0,30)$
At $x = 2$, $f(2) = 18$ minimum point of the function
The function has no inflection point as the second-order derivative ${f}''(x) = 6$ can never be zero and can never change the sign of the second-order derivative from positive to negative.

Using the above properties of $f(x) = 3x^{2} - 12x + 30$, we sketch the function in Figure 10.

(There are also a few functions in economics having this shape.)

Figure 10

Example 4

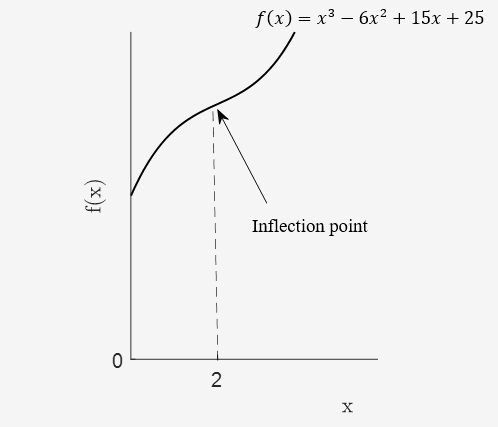
Given $f(x) = x^{3} - 6x^{2} + 15x + 25$, use the first-order derivative and the second-order derivative of the function to sketch the graph of the function.

Here, ${f}'(x) = 3x^{2} - 12x + 15$ and ${f}''(x) = 6x - 12$.

The first-order derivative of the given function ${f}'(x) = 3x^{2} - 12x + 15$ is always greater than zero.
The function is an increasing function on its entire domain.
For the function ${f}'(x) = 3x^{2} - 12x + 15 \neq 0$. Solving ${f}'(x) = 3x^{2} - 12x + 15 = 0$ applying the quadratic formula provides imaginary numbers. Therefore, the function has no critical point on its unrestricted domain or on any open interval of the domain.
However, the second-order derivative of the function evaluated at $x = 2$ is ${f}''(2) = 0$. At the interval of the domain lies on the left of $x = 2$ second-order derivative is always negative and at the interval of the domain lies on the right of the point $x = 2$, the second-order derivative is always positive. Therefore, $x = 2$ is the inflection point on the given function; concavity of the function changes at this point.
For example, at $x = 1$, ${f}''(1) = -6 < 0$ implies that the function is concave near the vicinity of $x = 1$. On the other hand, at ${f}''(3) = 6 > 0$ implies that the function is convex near the vicinity of $x = 3$. Therefore, in between these intervals, there exist a point where the concavity of the function changes. The second-order derivative ${f}''(2) = 0$ confirms that $x = 2$ is the point of inflection.
Evaluating the function at $x = 0$, and at the inflection point, provide useful information to sketch the graph:
At $x = 0$, $f(x) = 0 + 0 + 0 + 25 = 25$ vertical intercept $(0,25)$
At $x = 2$, $f(2) = 39$ at the inflection point f(x) = 39
$f(x) = x^{3} - 6x^{2} + 15x + 25$ is a function which is always increasing. Its vertical intercept is $25$.

In the interval $(0,2)$ The function is concave to the origin. Its inflection point is at $x = 2$. In the interval $(2,+\infty)$, the graph is convex to the origin. The graph is similar like the short-run total cost functions in economics. See Figure 11.

Figure 11

Example 5:

(Examples 1-4 discuss the answers in detail so that the readers can understand the concepts thoroughly. Example 5 presents a formal approach to deal with questions regarding optimization.)

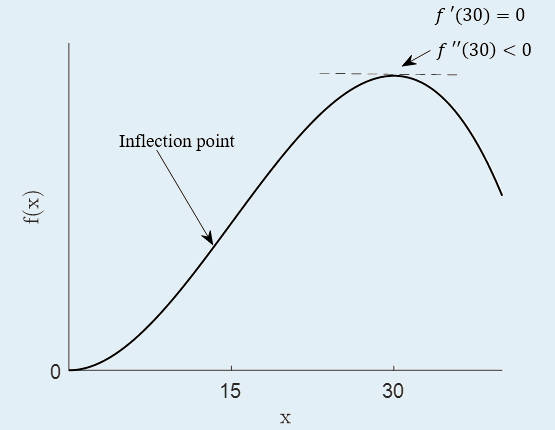
For the following function, (1) find the critical values, (2) test for local maxima and minima, (3) check for the inflection points, if any, and (4) sketch its graph. $$f(x) =45x^{2} - x^{3}$$ Answer:

Use the first-order condition to find the critical values: $${f}'(x) = 90x - 3x^{2} = 0$$ $$\qquad\quad\; 3x(30 - x) = 0$$
$x = 0$ $x = 30$ critical values
Using the second-order derivative, test for the local maxima and minima: $${f}''(x) = 90 - 6x$$ Evaluate ${f}''(x)$ at the critical values:
${f}''(0) = 90 > 0$ convex, local minima
${f}''(30) = -90 < 0$ concave, local maxima

At $x = 0$ the function is at the local min and at $x = 30$ is at the local max.
As the function is convex at the neighbourhood of $x = 0$ and concave at the vicinity of $x = 30$, in between these two points there exist an inflection point where the concavity changes. To find the inflection point, set the second-order derivative equals to zero and solve it: $${f}''(x) = 90 - 6x = 0$$ $$x =15 \qquad\;$$

There exists an inflection point at $x = 15$.
The graph of the function $f(x) = 45x^{2} - x^{3}$ is drawn in Figure 12.